The developed model is based on a classical SEIR model, which in mathematical epidemiology describes the spread of infections within a population. In this classical model, an individual can pass through four disease-relevant states:
- State S: Individuals who can be infected
- State E: Individuals who are infected, are infectious, but have not yet been identified as infected
- State I: Infected individuals
- State R: Recovered individuals.
The further developed SEIR/D model describes more complex relationships. In addition to the states S, E and R, infected people are subdivided into
- State C: Infected individuals who remain ambulatory
- State KH: Infected in hospital
- State ICU: Infected in intensive care unit
- State ICU ventilated: Infected persons requiring mechanical ventilation
In addition, the model was extended by state D: Infected people who have died. Likewise, state R was subdivided into
- State KH R: patients recovered during their stay in hospital and
- State R: recovered individuals outside the hospital
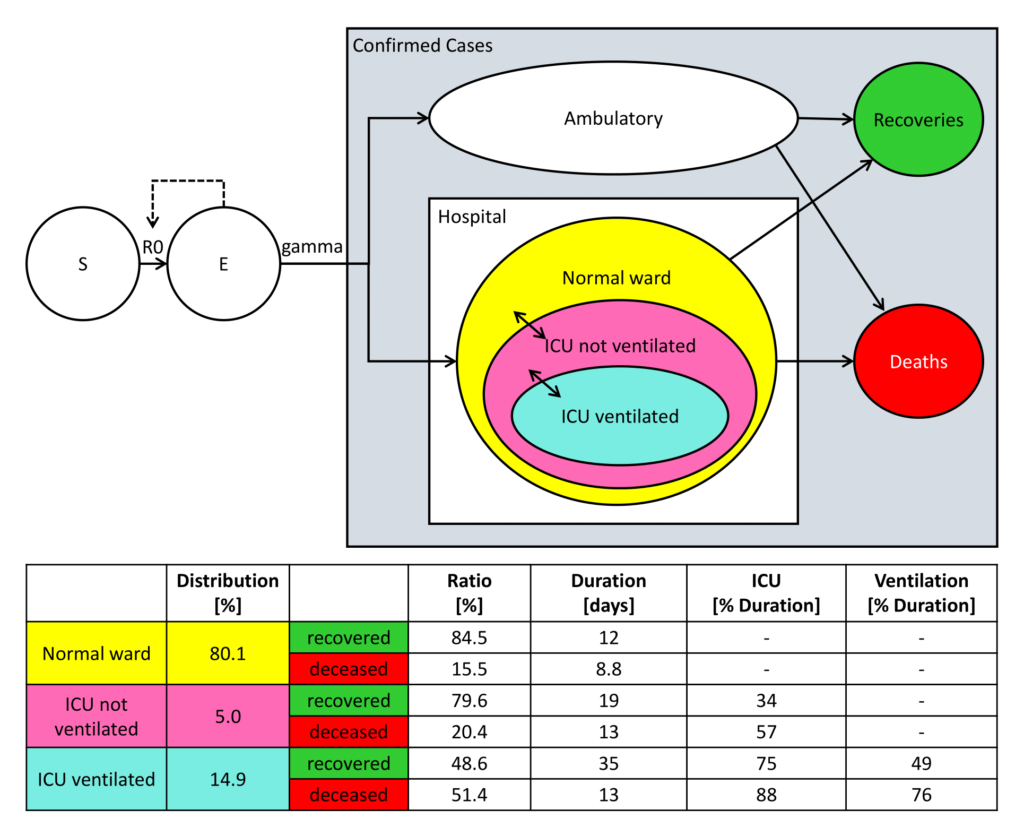
People from state E infect people from state S. The factor R0 (basic reproduction number) indicates how many people from state S are infected on average by a single person from state E. Infected people in state E are only identified as infected after a certain time (gamma) and thus reach state C (cases = confirmed cases). Infected persons (C) can either be recovered on an outpatient basis (R) or admitted to hospital (KH). Inpatients can recover in hospital (KH R), die in hospital (D: Death) or be transferred to intensive care (ICU). Infected patients in intensive care units (ICU) can also recover (KH R), die (D) or require mechanical ventilation (ICU ventilated).
The model structure with the different states as well as their transitions is shown in Fig. 1 The given data (length of stay, percentage share, ventilation, etc.) originate from hospital data of more than 30,000 German COVID-19 patients of more than 250 hospitals, which were derived anonymously from the MetaKIS system.